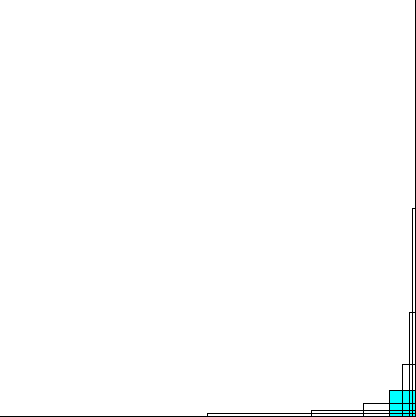
theorem & proof: the uniqueness

 of the absolute dead center cube

Theorem: That the 4-dimensional hypercube volume whose edge is the volume/surface ratio of a base cube is to the base cube volume exactly as the boundary of the 4-dimensional hypercube of the base cube is to its volume — (x/6)⁴/x³ = 8x³/x⁴ — is uniquely true of the cube whose edge is 10368^1/2 (the “absolute dead center cube”¹).

Proof: The ratio of volume to surface for all cubes where x is the edge length is x/6.  It is uniquely true for the cube whose edge is 10368^1/2 that (x/6)⁴ is at once the sum of its surface area + the area of its horizontal and vertical planes.²  36² and 8 are factors of 10368.  For all cubes (x/6)⁴/x³ × 8x³/x⁴ = 162⁻¹.  With x = 1, the value of (x/6)⁴/x³ = 36^-2.  The value of (x/6)⁴/x³ increases as the value of x increases to infinity, at the rate of 36^-2 per unit increase.   With x = 1, the value of 8x³/x⁴ = 8.  The value of 8x³/x⁴ decreases as the value of x increases to infinity, at the rate equal to the 8⁻¹ per unit increase in its inverse value.  (x/6)⁴/x³ = 8x³/x⁴ will then be true once, when x = 10368^1/2 –– when (x/6)⁴/x³ = 10368^1/2 × 36^-2 = 8x³/x⁴ = (10368^1/2/8)⁻¹.

Corollary I: Since 162 is the square of the volume/surface ratio of the absolute dead center hypercube, it is evident that all three-dimensional cubicity is constitutionally directed toward the mathematics of the fourth dimension and is so directed with perfect specificity to that of one particular hypercube.

Corollary II: Since it is the case that for all cubes (x/6)⁴/x³ × 8x³/x⁴ = 162⁻¹, and uniquely for the absolute dead center cube that [(x/6)⁴/x³]² = (8x³/x⁴)² = 162⁻¹, the latter is to the former as proportionate division in extreme and mean ratio is to proportionate division in all other ratios.³

Corollary III: Since for the absolute dead center cube [(x/6)⁴/x³]⁴ = (8x³/x⁴)⁴ = 162^-2, it is constitutionally uniquely fourth-dimensional—constitutionally perfectly hypercubic.  All other cubes are imperfectly so––constitutionally hyperrectangular, that is, for them (x/6)⁴/x³ ≠ 8x³/x⁴.

Note 1: The hypercubic rational constitution of the absolute dead center cube interrupts, once and for all, at a particular place, the infinite continuum of cubes hither and thither that place, for each of which it is true that its rational constitution is hyperrectangular.  Stated as a proportion, the rationally hypercubic constitution of the absolute dead center cube : the rationally hyperrectangular constitution of all other cubes :: fourth dimensional infinite flatness : second dimensional finite flatness :: the squaring of the squared rectangle : the squaring of the not-squared rectangle.

The infinite approach of hyperrectangular rational cubic constitutions to the hypercubic rational constitution of the absolute dead center cube is to their infinite recession thereafter as breadth is to depth, forming a hyperlinear angle whose vertex/diagonal is the hypercubic rational constitution of the absolute dead center cube.  Let the relation of squaring the squared rectangle to squaring the not-squared rectangle be reduced to the relation of square to rectangle, then there is a finitely flat, abstract image of the relation of the constitutional two-dimensional to four-dimensional flatness, the hypercubic rational constitution of the absolute dead center cube at once the perfect discontinuity of the continuum of hyperrectangular rational cubic constitutions, here illustrated:

Note 2: The Plot for (x/6)⁴/x³ = 8x³/x⁴, {x/1296, 8/x}, is:⁴

Notes

¹ D.G. Leahy, Foundation: Matter the Body Itself (Albany, 1996), Section III.5, pp. 433ff.
² (x/6)⁴ = 82944 = 8x² = 62208 + 20736, the area of the unitary structure of the absolute dead center cube (ibid.).  For the purely numerical uniqueness of 82944, cf. ibid., Sections III.6, III.7, and IV.2, p. 524, n. 98.  See also, on this web, Measure Beyond Beyond Reach, and The Magnitude of Being.
³ Note that, where φ is the division in extreme and mean ratio, the definition of the absolute dead center cube, (x/6)⁴/x³ = 8x³/x⁴ (= √162⁻¹), =  [(φ×10¹⁶²)^1/162]^{1/[162×(√162/2)2]}/10 × π/4 accurate to 1 part in 100 million.  See, on this web, The Beautiful Rationality of Π, √2, & Φ.
⁴ Adapted from WolframAlpha (http://www.wolframalpha.com/input/?i=%28x%2F6%29^4%2Fx^3+%3D+8x^3%2Fx^4).  Click on the image to see that when the x and y scales are equalized the circumference of the circle intersecting the (x/6)⁴/x³ = 8x³/x⁴ hyperbola = the Einstein field equation ratio of the curvature of spacetime to its matter/energy content, 8π = G_μν/T_μν.